Beschouw het onderstaande netwerk met vier pagina's.
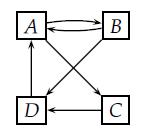
Bepaal welke webpagina het belangrijkst is.
Bepaal welke webpagina het belangrijkst is.
Antwoord 1 correct
Correct
Antwoord 2 optie
pagina B
Antwoord 2 correct
Fout
Antwoord 3 optie
pagina C
Antwoord 3 correct
Fout
Antwoord 4 optie
pagina D
Antwoord 4 correct
Fout
Antwoord 1 optie
pagina A
Antwoord 1 feedback
Correct: De overgangsmatrix ziet er als volgt uit.
$$\begin{equation}
G=\begin{pmatrix}
0 & \frac{1}{2} & 0 & 1\\
\frac{1}{2} & 0 & 0 & 0\\
\frac{1}{2} & 0 & 0 & 0\\
0 & \frac{1}{2} & 1 & 0\\
\end{pmatrix}
\end{equation}$$
De uitgebreide matrix van $G-I$ geeft onderstaande matrix.
$$\begin{equation}
\begin{pmatrix}
-1& \frac{1}{2} & 0 & 1&|& 0\\
\frac{1}{2} & -1 & 0 & 0&|& 0\\
\frac{1}{2} & 0 & 0 & 0& | & 0\\
0 & \frac{1}{2} & 1 & 0 & | & 0 \\
\end{pmatrix}
\end{equation}$$
Vegen geeft onderstaande matix.
$$\begin{equation}
\begin{pmatrix}
1 & 0 & 0 & -\frac{4}{3} &|& 0\\
0 & 1 & 0 & -\frac{2}{3} &|& 0\\
0 & 0 & 1 & -\frac{2}{3} &| & 0\\
0 & 0 & 0 & 0 &|&0\\
\end{pmatrix}
\end{equation}$$
Dit geeft $x_1=\frac{4}{3}x_4$, $x_2=x_3=\frac{2}{3}x_4$ en samen met $x_1+x_2+x_3+x_4=1$ geeft dit het onderstaande evenwicht.
$$\begin{equation}
\begin{pmatrix}
\frac{4}{11}\\
\frac{2}{11}\\
\frac{2}{11}\\
\frac{3}{11}\\
\end{pmatrix}
\end{equation}$$
Dus A is de belangrijkste pagina.
$$\begin{equation}
G=\begin{pmatrix}
0 & \frac{1}{2} & 0 & 1\\
\frac{1}{2} & 0 & 0 & 0\\
\frac{1}{2} & 0 & 0 & 0\\
0 & \frac{1}{2} & 1 & 0\\
\end{pmatrix}
\end{equation}$$
De uitgebreide matrix van $G-I$ geeft onderstaande matrix.
$$\begin{equation}
\begin{pmatrix}
-1& \frac{1}{2} & 0 & 1&|& 0\\
\frac{1}{2} & -1 & 0 & 0&|& 0\\
\frac{1}{2} & 0 & 0 & 0& | & 0\\
0 & \frac{1}{2} & 1 & 0 & | & 0 \\
\end{pmatrix}
\end{equation}$$
Vegen geeft onderstaande matix.
$$\begin{equation}
\begin{pmatrix}
1 & 0 & 0 & -\frac{4}{3} &|& 0\\
0 & 1 & 0 & -\frac{2}{3} &|& 0\\
0 & 0 & 1 & -\frac{2}{3} &| & 0\\
0 & 0 & 0 & 0 &|&0\\
\end{pmatrix}
\end{equation}$$
Dit geeft $x_1=\frac{4}{3}x_4$, $x_2=x_3=\frac{2}{3}x_4$ en samen met $x_1+x_2+x_3+x_4=1$ geeft dit het onderstaande evenwicht.
$$\begin{equation}
\begin{pmatrix}
\frac{4}{11}\\
\frac{2}{11}\\
\frac{2}{11}\\
\frac{3}{11}\\
\end{pmatrix}
\end{equation}$$
Dus A is de belangrijkste pagina.
Antwoord 2 feedback
Antwoord 3 feedback
Antwoord 4 feedback