Definition: A function of the form
z(x,y)=min{ax,by},
where a>0 and b>0 is called a minimum function.
Remark: The function value is determined by the the element (ax or by) with the smallest value. Therefore, we can rewrite the function as
z(x,y)={axif~ax≤by,byif~ax>by.
Example: The function z(x,y)=min{3x,4y} is an example of a minimum function.
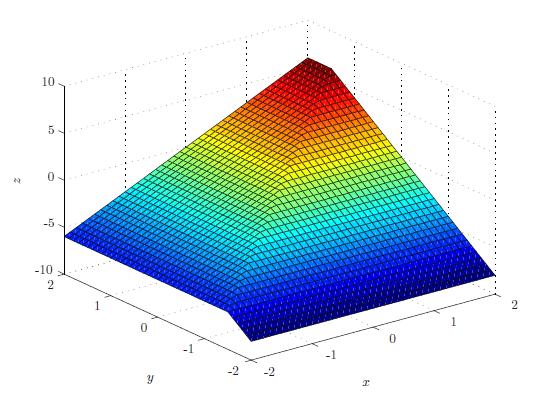
z(x,y)=min{ax,by},
where a>0 and b>0 is called a minimum function.
Remark: The function value is determined by the the element (ax or by) with the smallest value. Therefore, we can rewrite the function as
z(x,y)={axif~ax≤by,byif~ax>by.
Example: The function z(x,y)=min{3x,4y} is an example of a minimum function.


