Which of the following graphs depicts the level curves of z(x,y)=x2+y2 with values k=1 and k=4?
Antwoord 1 correct
Correct
Antwoord 2 optie
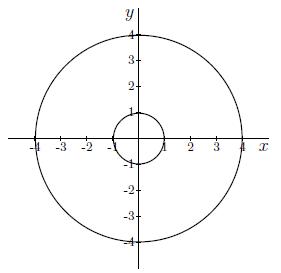
Antwoord 2 correct
Fout
Antwoord 3 optie
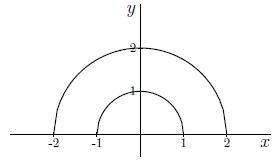
Antwoord 3 correct
Fout
Antwoord 4 optie
It is not possible to draw the level curves of this function, because z(x,y)=k cannot be rewritten.
Antwoord 4 correct
Fout
Antwoord 1 optie
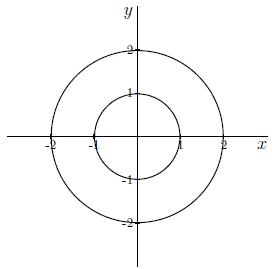
Antwoord 1 feedback
Correct: Rewriting of x2+y2=k for k=1 gives:
x2+y2=1⟺y2=1−x2⟺y=±√1−x2.
The graph of this is the central circle; you might recognize x2+y2=1 as the circle equation with center (0,0) and radius √1=1. Rewriting of x2+y2=k for k=4 gives:
x2+y2=4⟺y2=4−x2⟺y=±√4−x2.
The graph of this is the outside circle; you might recognize x2+y2=4 as the circle equation with center (0,0) and radius √4=2.
Go on.
x2+y2=1⟺y2=1−x2⟺y=±√1−x2.
The graph of this is the central circle; you might recognize x2+y2=1 as the circle equation with center (0,0) and radius √1=1. Rewriting of x2+y2=k for k=4 gives:
x2+y2=4⟺y2=4−x2⟺y=±√4−x2.
The graph of this is the outside circle; you might recognize x2+y2=4 as the circle equation with center (0,0) and radius √4=2.
Go on.
Antwoord 2 feedback
Antwoord 3 feedback

